...
The equations that we will use look as follows:
Conservation of mass:
| Latex |
|---|
| Wiki Markup |
{latex} \begin{equation*} \frac{\partial \rho}{\partial t}+\nabla \cdot \rho \vec{v}^{\,}_r =0 \end{equation*} {latex} |
Conservation of Momentum (Navier-Stokes):
| Latex |
|---|
| Wiki Markup |
{latex} \begin{equation*} \nabla \cdot (\rho \vec{v}^{\,}_r \vec{v}^{\,}_r)+\rho(2 \vec{\omega}^{\,} \times \vec{v}^{\,}_r+\vec{\omega}^{\,} \times \vec{\omega}^{\,} \times \vec{r}^{\,})=-\nabla p +\nabla \cdot \overline{\overline{\tau}}_r \end{equation*} {latex} |
Where
| Wiki Markup |
|---|
| Latex |
{latex}$\vec{v}^{\,}_r${latex} |
| Wiki Markup |
|---|
| Latex |
{latex}$\vec{\omega}^{\,}${latex} |
Note the additional terms for the Coriolis force (
| Wiki Markup |
|---|
| Latex |
{latex}$2 \vec{\omega}^{\,} \times \vec{v}^{\,}_r${latex} |
| Wiki Markup |
|---|
| Latex |
{latex}$\vec{\omega}^{\,} \times \vec{\omega}^{\,} \times \vec{r}^{\,}${latex} |
| Wiki Markup |
|---|
| Latex |
{latex}$\vec{\omega}^{\,}= -2.22 \mathbf{\hat{k}}${latex} |
For more information about flows in a moving frame of reference, visit ANSYS Help View > Fluent > Theory Guide > 2. Flow in a Moving Frame of Reference and ANSYS Help Viewer > Fluent > User's Guide > 9. Modeling Flows with Moving Reference Frames.
...
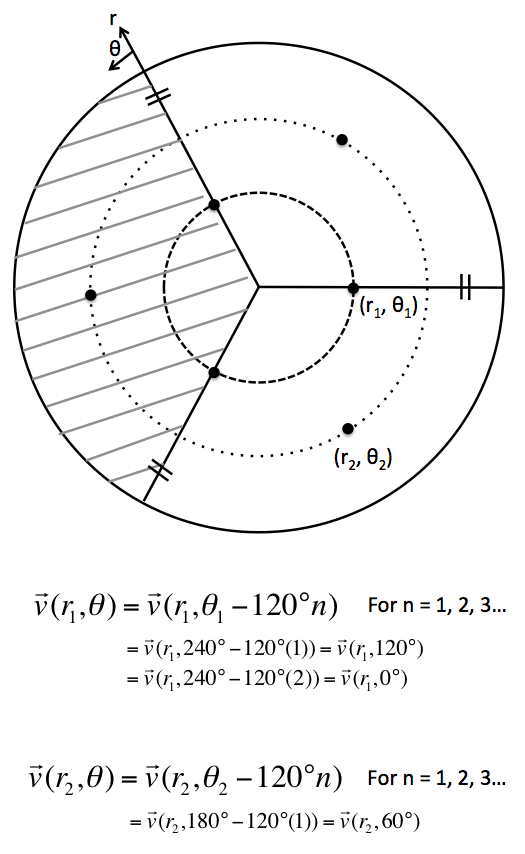
We model only 1/3 of the full domain using periodicity assumptions:
| Latex |
|---|
| Wiki Markup |
{latex} \begin{equation*} \vec{v}^{\,}(r_1,\theta) = \vec{v}^{\,}(r_1,\theta_1 - 120n) \end{equation*} {latex} |
This therefore proves that the velocity distribution at theta of 0 and 120 degrees are the same. If we denote theta_1 to represent one of the periodic boundaries for the 1/3 domain and theta_2 being the other boundary, then
| Wiki Markup |
|---|
| Latex |
{latex}$\vec{v}^{\,}(r_i,\theta_1)=\vec{v}^{\,}(r_i,\theta_2)${latex} |
The boundary conditions on the fluid domain are as follow:
...
The velocity, v, on the blade should follow the formula
| Latex |
|---|
| Wiki Markup |
{latex} \begin{equation*} v=r \times \omega_{} \end{equation*} {latex} |
Plugging in our angular velocity of -2.22 rad/s and using the blade length of 43.2 meters plus 1 meter to account for the distance from the root to the hub, we get
| Latex |
|---|
| Wiki Markup |
{latex} $$v=-2.22\ \mathrm{rad/s}\ \mathbf{\hat{k}} \times -44.2\ \mathrm{m}\ \mathbf{\hat{i}}$$ $$v=98.10\ \mathrm{m/s}\ \mathbf{\hat{j}}$$ {latex} |
Additionally, by using the simple one-dimensional momentum theory, we can estimate the power coefficient which is the fraction of harnessed power to total power in the wind for the given turbine swept area. This analysis uses the following assumptions:
...
Thus, at rated wind speed,
| Latex |
|---|
| Wiki Markup |
{latex} \begin{eqnarray*} C_p = \frac{P_{rated}}{P_{wind}} = \frac{P_{rated}}{0.5\rho A V_{rated}^3} = \frac{P_{rated}}{0.5(1.225\frac{kg}{m^3})(\frac{\pi(82.5m)^2}{4})(11.5\frac{m}{s})^3} = 0.30 \end{eqnarray*} {latex} |
The resulting power coefficient of 0.30 is very reasonable. We will compare it to power coefficient obtained from the simulation in the Verification & Validation section.
...
 Sign-up for free online course on ANSYS simulations!
Sign-up for free online course on ANSYS simulations!