Before we explore the ANSYS results, let's take a peek at the mesh.
Click on Mesh (above Solution ) in the tree outline. This shows the mesh used to generate the ANSYS solution. The domain is a rectangle. This domain is discretized into a number of small "elements". Recall that ANSYS solves the BVP and calculates the displacements at the nodes. A finer mesh is used near the left and right ends where we expect greater stress concentration. We have checked that the solution presented to you is reasonably independent of the mesh.
Set the units for the results display by selecting Units > Metric (mm, kg, N, s, mV, mA) . The displacements will be reported in mm and the stresses in N/mm2 which is equivalent to MPa.
To view the deformed structure, click on Solution > Displacement in the tree outline. The black rectangle shows the undeformed structure. The deformed structure is colored by the magnitude of the displacement. The displayed displacement distribution is calculated by interpolating the nodal displacements. Red areas have deformed more and blue areas less. You can see that the left end has not moved as specified in the problem statement. This means this boundary condition has been applied correctly. The displacement increases from left to right as we intuitively expect. There is also not much variation in the y-direction. So we can conclude that the model has been constrained properly.
Note the extremely high deformation near the point load. This extremum is unrealistic and should be ignored (there are no point loads in reality).

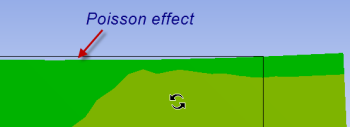
To view the Poisson effect (shrinking in the y direction), zoom into the top-rightright corner by drawing a rectangle around the region with the right mouse button.

You can do this multiple times to zoom in more. You do indeed see the shrinking in the y-direction as expected but it is small for this model.
You can restore the front view of the entire model by right-clicking in the background and choosing View > Front .
Note that you can zoom in and out using the middle mouse wheel. You can translate the model by clicking on the Pan button and dragging the model with the left mouse button. There are also a bunch of zoom options next to the Pan button.
The stresses are derived from the nodal displacements using Hooke's law. In the following video, we look at the sigma_x distribution in the interior and at the boundaries and compare the ANSYS values to the values expected from the analytical solution and traction boundary conditions.
<iframe width="600" height="338" src="//www.youtube.com/embed/vBNFUYrsWMw?rel=0" frameborder="0" allowfullscreen></iframe> |
Summary of the above video investigating sigma_x:
In the video, we saw that ANSYS's values for sigma_x matches with:
Next, let's take a look at sigma_y. Click on Solution > sigma_y in the tree outline. Again, probe values in the middle as well as at the ends. Check that:

We expect tau_xy to be zero away from the ends. Near the ends, since sigma_x and sigma_y are non-zero, we expect
\begin{align*}
\tau_{xy} = \tau_{xy}(x,y)
\end{align*} |
Plot tau_xy, look at the range of values and use Probe to check actual values. Are the above statements valid?

The Equivalent or Von Mises stress is used to predict yielding of the material. We can see that the analytical solution under-predicts the maximum equivalent stress. Thus, one would need to use a large factor of safety if using the analytical result while designing such a structure. One would use a factor of safety with the FEA result also but it does not have to be as large.

Go to Step 3: Verification and Validation
Go to all ANSYS Learning Modules